Sloane and Plouffe 1995, p. 33 Hardy 1999, p. 46), it requires special
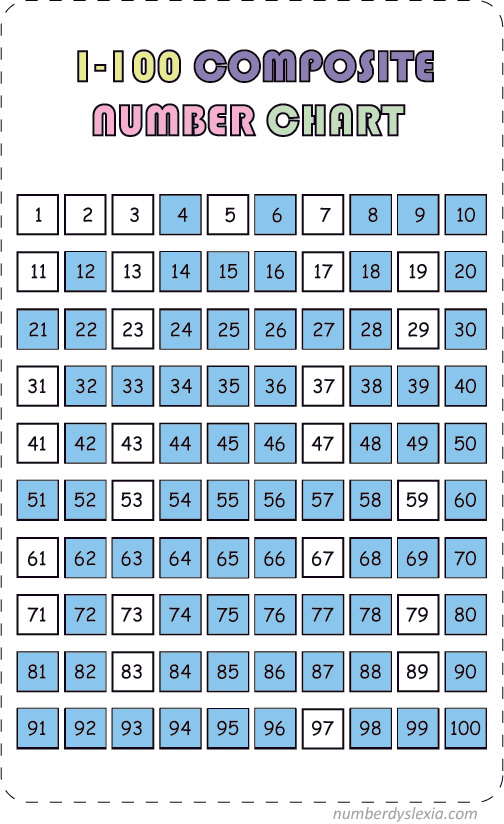
Although the number 1 used to be considered a prime (Goldbachġ742 Lehmer 1909, 1914 Hardy and Wright 1979, p. 11 Gardner 1984, pp. 86-87 The number 1 is a special case which is considered neither prime nor composite (Wells 1986, p. 31). While the term 'prime number' commonly refers to prime positive integers, other types of primes are also defined, such as the Gaussian Integers other than 1 which are not prime are called composite For example, the only divisors ofġ3 are 1 and 13, making 13 a prime number, while the number 24 has divisors 1, 2,ģ, 4, 6, 8, 12, and 24 (corresponding to the factorization ), making 24 not a prime number. Meaning it is a number that cannot be factored. Having exactly one positive divisor other than 1, More concisely, a prime number is a positive integer A prime number (or prime integer, often simply called a 'prime' for short) is a positive integer that has no positive integer divisors